As we have said in numerous articles, the advantage of a casino/player is one of the fundamental indicators by which you should measure individual bets. It tells us how much one participant is in an advantage over the other. Of course, your goal is to choose those bets/casino games that have the house edge as close to zero as possible or even flip into the player's advantage.
Tip: Learn more about probability and payout of slots. Use our now slot machine calculator and find out which slots are favourable.
This is what the expected value is for us. Mathematically, it is a weighted average:
EV = X1P1 + X2P2…. + XnPn
EV = Expected Value
X = situation result (profit / loss)
P = probability for individual situations
Coin Flip
Of course, this is best explained in an example. We will, therefore, use the basic coin flip. There will be two players who will bet one coin on one side of the coin. The player who guesses the result takes both coins. This is a 1 : 1 bet. If we convert our assignment into the equation above, we get:
EV = 1 x 0.5 + (-1) x 0.5
EV = 0
Thus, in the equation, we can see two situations that may occur. First of all, the goal here is to guess which side of the coin will fall and win one coin (1 x 0.5). Or, you will lose the bet (-1 x 0.5).
The expected value, in this case, is 0% and this is an even bet. It means that the longer these players flip coins, the closer their total profit or loss will be to zero.
The Expected Value for Roulette
In casinos, however, there are very few casino games with zero house edge. The best way to calculate the casino advantage is on roulette bets because it has the same value is based on all bets. Specifically, 2.7% for European roulette and 5.26% for American roulette. According to the formulas:
European Roulette; colour bet - payout 1 : 1, the chance of winning 18/37, the chance of losing 19/37
EV = (1 x 18/37) + (-1 x 19/37) = -0.02703 => -2.703%
American Roulette; colour bet - payout 1 : 1, the chance of winning 18/38, the chance of losing 20/38
EV = (1 x 18/38) + (-1 x 20/38) = -0.05263 => -5.263%
We came with the results the same way as we did for coin flips. 1 and -1 are winning and losing, and the probability is calculated by the number of winning and non-winning fields. Let us look now at how the expected value will be reflected in the adoption of the Le Partage rule, which can be seen on some roulettes in Monte Carlo or other European cities. This rule states that if a player bets an even bet and a 0 falls, half of the bet is returned, and the other forfeits to the casino.
EV = (1 x 18/37) + (-1 x 18/37) + (-0.5 x 1/37) = -0.0135
The casino advantage is reduced to 1.35% in this case. But beware! This rule applies only to even bets. For other bets, the traditional value remains 2.7%.
Combined Bets in Roulette
However, roulette rules allow the basic bets to be combined to cover any part of the field. Some roulette betting systems are even based on this principle. We will now discuss how the expected value for such combined bets will be calculated.
Consider a somewhat more manageable situation where you bet one chip on three bets:
- One chip on red
- One chip for the first dozen
- One chip for number one
In the picture, you can see the bets placed and the number of winning fields. So what can happen:
- Number 1 will fall - We win all 3 bets, totaling 38 coins (35 + 2 + 1). Probability 1/37
- Red from the first dozen - We win a total of two coins (2 + 1-1). Probability 5/37
- Black falls in the first dozen - We win zero coins (2-1-1). Probability 6/37
- Red falls in second or third dozen - We lose one coin (1-1-1). Probability 12/37
- Black falls in second or third dozen or 0 - We lose three coins (-1-1-1). Probability 13/37
The resulting formula will look like this:
EV = (38 x 1/37) + (2 x 5/37) + (0 x 6/37) + (-1 x 12/37) + (-3 x 13/37) = -0.08108
The result can then be interpreted as a loss of 0.081 coins for every this particular bet, which is exactly 2.7% from the three coins placed on this bet. So there is a mathematical proof that betting systems using different gaming coverage to raise the house edge of roulette simply do not work.
The Expected Value for Other Casino Games
Using the same principle as described for roulette, you can then calculate the expected value for different bets. Let's first look at the game of blackjack. The house edge of blackjack itself is difficult to determine, and as the casino's advantage always reflects the rules of each table, it is a variable number. However, this does not limit us from calculating the expected value for one of its side bets.
So let's try to analyze the Perfect pair side bet that can be found in some casinos. As the name suggests, the bet is that the player creates a pair from the first two cards. For our analysis, let's say it's played with 6 decks.
| Hand | Payout | Odds |
| Mixed Pairs | 5 to 1 | 12/311 |
| Suited Pairs | 10 to 1 | 6/311 |
| Identical Pairs | 30 to 1 | 5/311 |
| No Pairs | -1 | 288/311 |
If we add the information from the table into our equation:
EV = (5 x 12/311) + (10 x 6/311) + (30 x 5/311) + (-1 x 288/311) = - 0.05787
The house edge for this side bet is 5.777%.
The Pass Bet at Craps
Because craps are a very popular game, we will also show you how to calculate the expected value for the most common craps bet - the Pass bet. The player wins if the shooter rolls 7 or 11 in the first round or shoots the number 4, 5, 6, 8, 9, 10 to set the point, and then hits that number again. This time we'll do it a little differently. First of all, it is necessary to know the individual combinations for the dice.
| Sum | Probability | Combinations |
| 2 | 1/36 | 1,1 |
| 3 | 2/36 | 1,2+2,1 |
| 4 | 3/36 | 1,3+3,1+2,2 |
| 5 | 4/36 | 1,4+4,1+2,3+3,2 |
| 6 | 5/36 | 1,5+5,1+2,4+4,2+3,3 |
| 7 | 6/36 | 1,6+6,1+2,5+5,23,4+4,3 |
| 8 | 5/36 | 2,6+6,2+3,5+5,3+4,4 |
| 9 | 4/36 | 3,6+6,3+4,5+5,4 |
| 10 | 3/36 | 4,6+6,4+5,5 |
| 11 | 2/36 | 5,6+6,5 |
| 12 | 1/36 | 6+6 |
Now, we can select probabilities from the table. A total of 36 different combinations can be thrown with two dice, if the number 7 can be rolled in a total of six ways, the probability for number 7 will be 6/36. According to the same logic, we determine the probabilities for the other numbers.
It is important to define the formula for a situation where a point is formed and the continues until the same number falls or 7 falls and the players lose the pass bet. By editing the formulas, we get the expression p / (p + q), where p is the probability for winning and q is the probability for 7. The formula will then look like:
- Probability for instant win (7 + 11) = 6/36 + 2/36 = 8/36
- Probability to win after setting point = pr4 x pr (4 before 7) + pr5 x pr (5 before 7) + pr6 x pr (6 before 7) + pr8 x pr (8 before 7) + pr9 x pr (9 before 7 ) + pr10 x pr (10 before 7)
- Probability to Win = 8/36 + (3/36) x (3/9) + (4/36) x (4/10) + (5/36) x (5/11) + (5/36) x (5/11) + (4/36) x (4/10) + (3/36) x (3/9) = 244/495
- Probability for Loss = 1 - (244/495) = 251/495
EV = (1 x 244/495) + (-1 x 251/495) = -0.014141
The house edge for the pass bet is 1.41%. You can then calculate all bets in the same way.
Even Bets
You may notice that all bets for which we have calculated the expected value in this article came out negative. Thanks to that, casinos make a profit in the long run. However, there are games where the player is at an advantage, and his expected value is so positive. One of those games is Wild Deuces video poker. In certain situations, card counters at blackjack also have advantages. In both cases, however, it is necessary to hold to the optimal strategy.
At the beginning of the coin roll article, we could see an example of a straight bet where neither the casino nor the player has the advantage. Again, we will return to roulette now and estimate how much the player would have to bet on the single number so that EV would 0. Still, we use the same equation, but instead of EV, we use 0, and instead of win, we use X.
0 = (X x 1/37) + (-1 x 36/37)
36/37 = X / 37
X = 36 coins
The estimation shows that if a casino paid out 36 coins for the single bet, the casino would not get any profit or loss in the long run on roulette.
Of course, you can also use these calculations for bets you don't find in a casino. Let's say you will bet on who takes the higher card from the card deck. Your opponent pulls out of 52 card deck card with value 9. You bet the one coin. The cards are ranked just like in poker, and the ace is always 11. How much you must win to make expected value equal to 0.
Chance to win = 20/51 (20 cards are worth more than 9, and only 51 cards remain in the deck)
Loss chance = 31/51
0 = (X x 20/51) + (-1 x 31/51)
X = 1.55 coin
In the case described above, if you do not get at least 1.55 coin for every coin betted, you are playing with a negative expectation.
Following precisely the same procedure, you can then calculate the expected value of practically anything that can be calculated with a probability, and the result is a profit or loss. It is important to remember when creating formulas that the sum of probabilities must always be 1.

 EN
EN  čeština
čeština  Polski
Polski  Slovenčina
Slovenčina  Русский
Русский  Deutsch
Deutsch  Slovenski
Slovenski  Dutch
Dutch  Svenska
Svenska  Português
Português  Italiano
Italiano  Español
Español  Français
Français  Suomalainen
Suomalainen  Български
Български  Românesc
Românesc  Magyar
Magyar  Eesti
Eesti  Ελληνική
Ελληνική  Latviešu
Latviešu  Lietuvių
Lietuvių  やまと
やまと  中国
中国  Dansk
Dansk  Türkçe
Türkçe  ID
ID  Українська
Українська  한국어
한국어  Norsk
Norsk  Peter Sommer
Peter Sommer 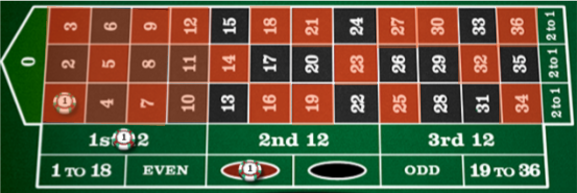






You have to be logged in to add a comment